Selected: Edexcel A Level Maths - Pure Maths
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
OCR MEI H630/02 Jun 2023 AS Exam Q. 4 : 3 marks in 3:51 min.
OCR MEI H640/03 Jun 2022 A2 Exam Q. 1 : 2 marks in 3:12 min.
OCR MEI H630/01 Jun 2022 AS Exam Q. 4 : 6 marks in 7:43 min.
OCR MEI H630/01 Jun 2022 AS Exam Q. 6 c : 3 marks in 3:52 min.
OCR MEI H640/03 Nov 2021 A2 Exam Q. 8 : 3 marks in 4:48 min.
OCR MEI H630/01 Nov 2021 AS Exam Q. 9 a : 4 marks in 5:09 min.
OCR MEI H630/01 Jun 2019 AS Exam Q. 11 : 11 marks in 14:09 min.
OCR MEI H630/01 Jun 2019 AS Exam Q. 7 : 11 marks in 14:09 min.
OCR MEI H640/02 Jun 2018 A2 Exam Q. 5 : 3 marks in 3:36 min.
OCR MEI H630/01 Jun 2017 AS Sample Exam Q. 10 : 12 marks in 15:26 min.
Edexcel 8MA0/01 Jun 2023 AS Exam Q. 4 : 5 marks in 6:00 min.
Edexcel 8MA0/01 Apr 2023 AS Mock Q. 4 c : 4 marks in 4:48 min.
Edexcel 8MA0/01 Jun 2022 AS Exam Q. 7 : 7 marks in 8:24 min.
Edexcel 9MA0/02 Mar 2020 A2 Mock Q. 8 : 9 marks in 10:48 min.
Edexcel 9MA0/01 Jun 2019 A2 Exam Q. 13 a : 3 marks in 3:36 min.
Edexcel 9MA0/01 Jun 2019 A2 Shadow Exam Q. 13 a : 3 marks in 3:36 min.
Edexcel 8MA0/01 Jun 2019 AS Exam Q. 7 : 8 marks in 9:36 min.
Edexcel 8MA0/01 Jun 2017 AS Sample Exam Q. 13 : 7 marks in 8:24 min.
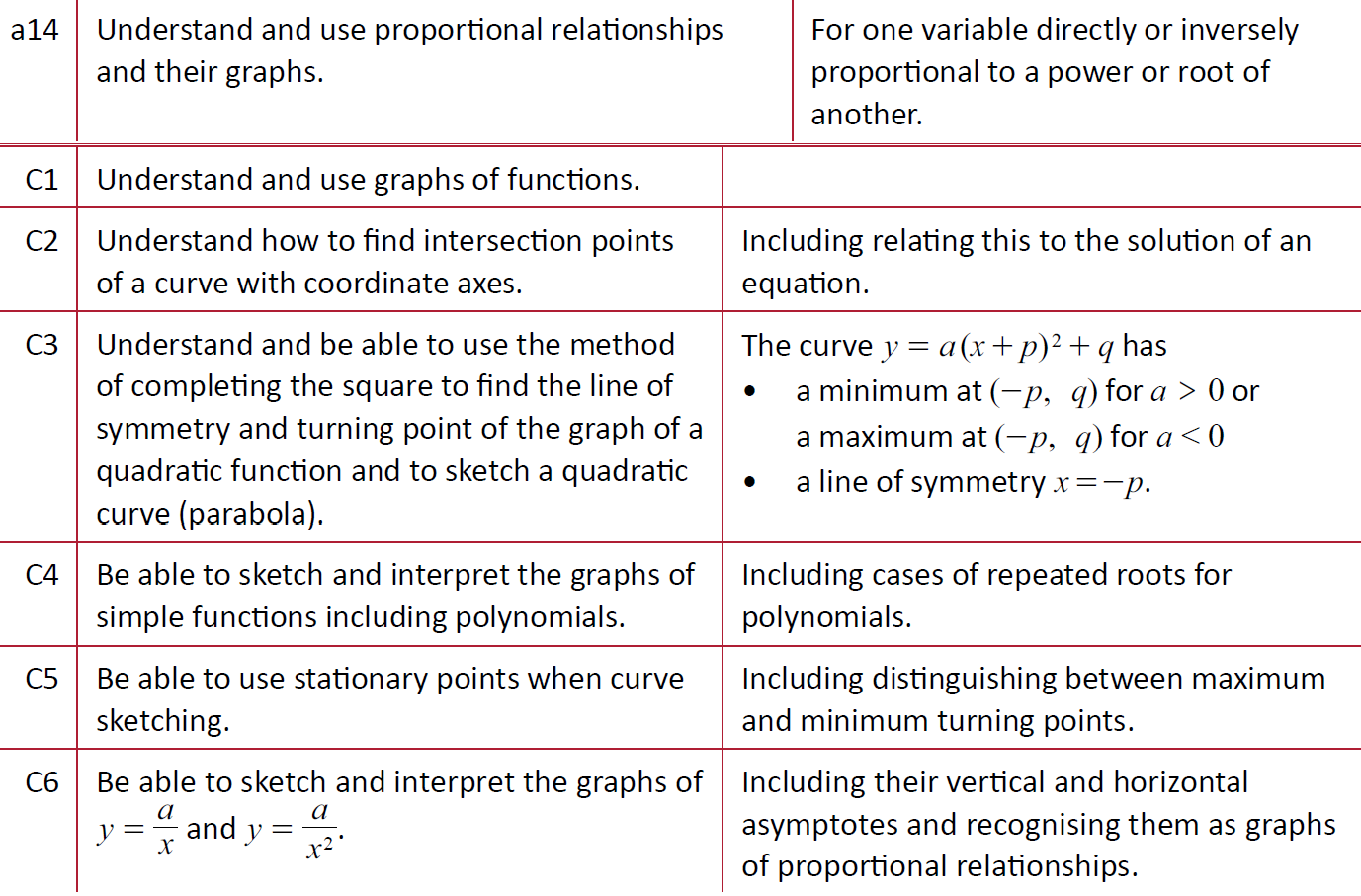
Explore the roots and intercepts of cubic graphs
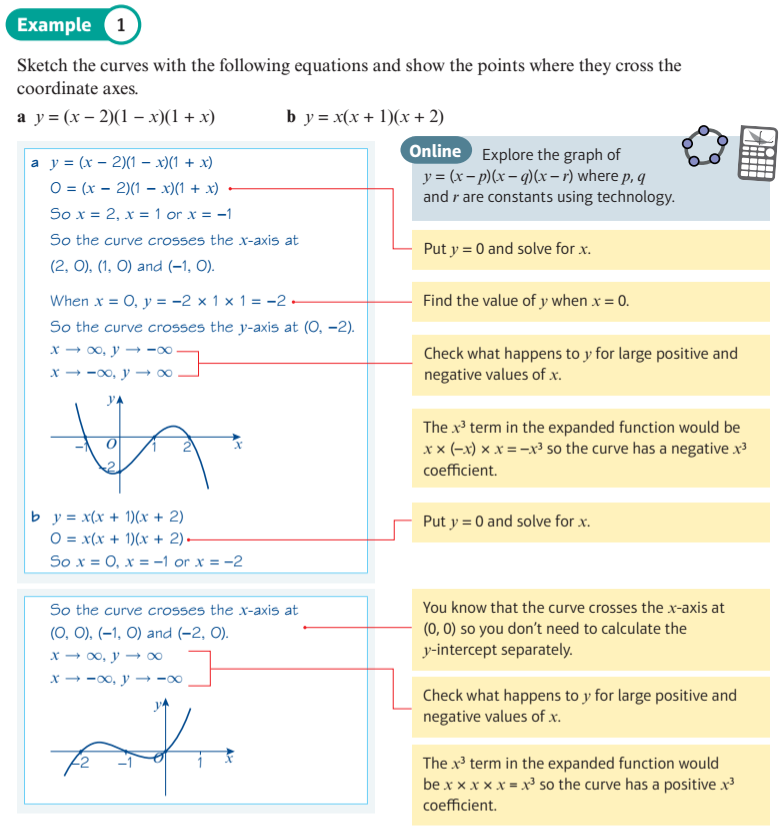
Explore the roots and shapes of quartic functions
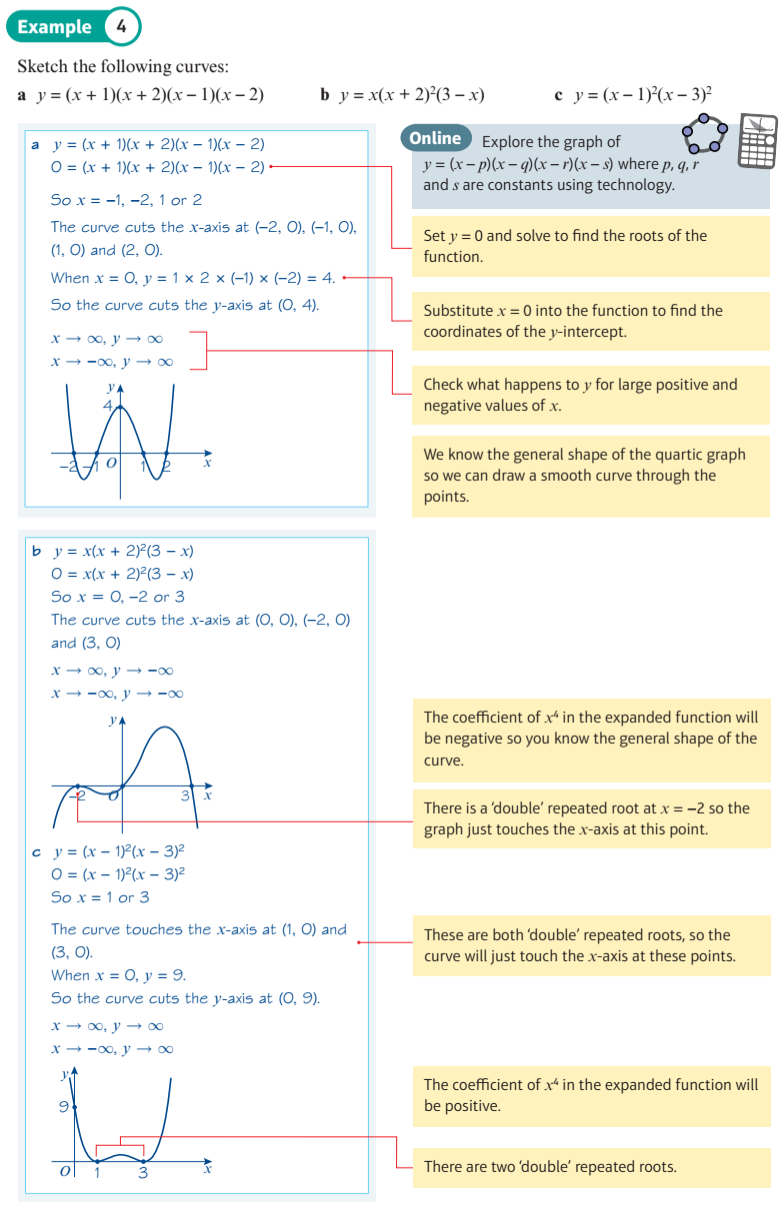
Explore reciprocal graphs
Matching Polynomials
You can select ten green polynomial curves on this page.
Selecting the order of the curve, and using the sliders to match the blue dashed curve to the green curve will reveal the equation in each case, but can you say what the equation is before making the match?
Selecting the order of the curve, and using the sliders to match the blue dashed curve to the green curve will reveal the equation in each case, but can you say what the equation is before making the match?
Coordinate Geometry - Rational Curves - y=(x-a)/(x-b)
The graph of $\color{blue}{y = { {x - a} \over {x - b} } }$
Change the values of $\color{blue}{a}$ and $\color{blue}{b}$ and observe the effect this has on the curve and its asymptotes.
Change the values of $\color{blue}{a}$ and $\color{blue}{b}$ and observe the effect this has on the curve and its asymptotes.
Coordinate Geometry - Asymptotes - Asymptotes of y=a/(x-b)
An asymptote is a line towards which a given curve is approaching but never meets. Asymptotes are often vertical or horizontal lines, but can also be at an angle.
The graph displayed is described by the Cartesian equation: $\color{blue}{y = {{a} \over {x - b}}}$.
Change the values of $\color{blue}{a}$ and $\color{blue}{b}$ and observe the effect this has on the curve and its asymptotes. What are the equations of these asymptotes?
The graph displayed is described by the Cartesian equation: $\color{blue}{y = {{a} \over {x - b}}}$.
Change the values of $\color{blue}{a}$ and $\color{blue}{b}$ and observe the effect this has on the curve and its asymptotes. What are the equations of these asymptotes?
Odd and Even Functions
Functions with the property that f(-a) = f(a) for all values of a are called even.
Functions with the property that f(-a) = -f(a) for all values of a are called odd.
Drag the sample point to help decide whether each of the 10 functions given below are odd, even or neither.
What geometric properties do odd and even functions have?
Functions with the property that f(-a) = -f(a) for all values of a are called odd.
Drag the sample point to help decide whether each of the 10 functions given below are odd, even or neither.
What geometric properties do odd and even functions have?