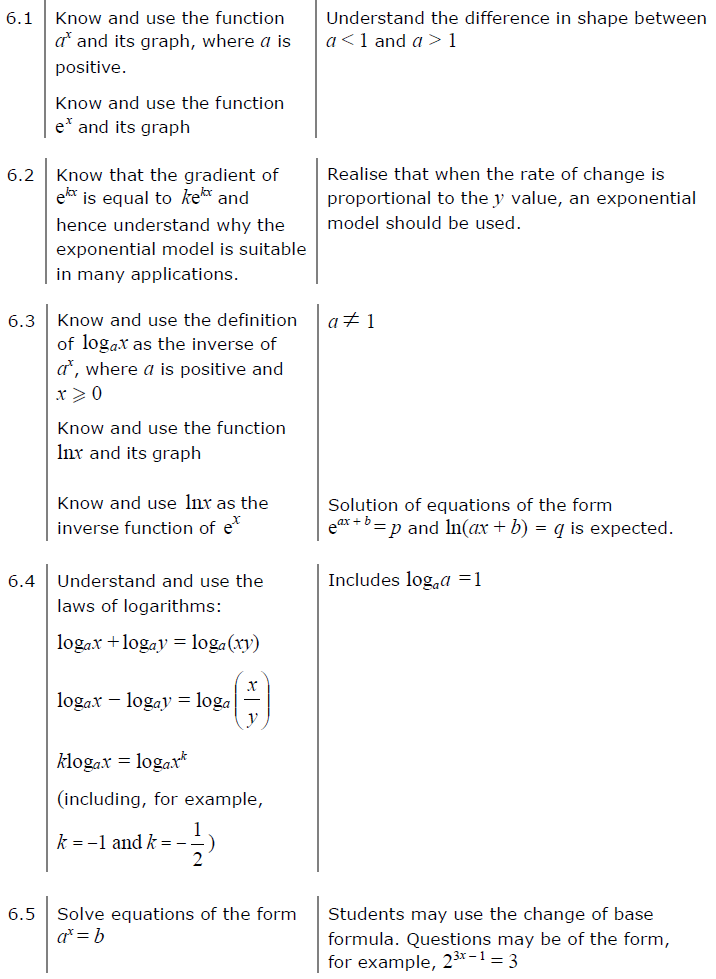

Using exponential functions
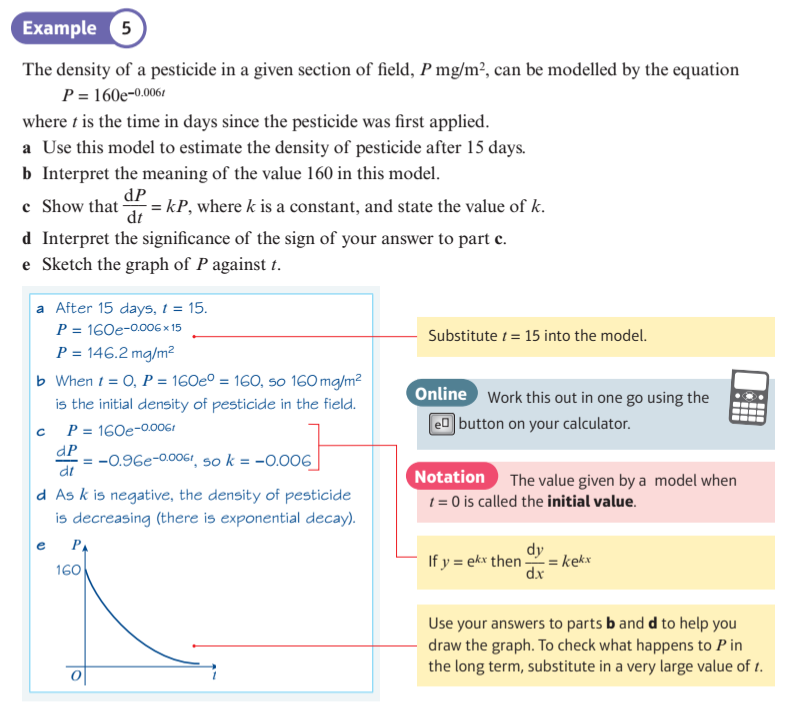
Using logarithms to any base
Explore relationship between exponential functions and their derivatives
Exponential Graphs: ${a^x}$
The graph of $\color{blue}{ f(x) = {a^x} }$ is shown in blue,
together with its tangent and gradient at a sample point in red.
By considering the gradient at x = 0, 1, 2, 3, can you predict what the gradient function of $\color{blue}{ f(x) = {a^x} }$ is?
By considering the gradient at x = 0, 1, 2, 3, can you predict what the gradient function of $\color{blue}{ f(x) = {a^x} }$ is?
Gradients of ${a^x}$ and ${e^x}$
Compare the graph of \(\color{blue}{ f(x) = {a^x} }\) with the graph of its gradient function, \(\color{green}{ f'(x) }\).
Adjust the value of a till the the two curves coincide.
Adjust the value of a till the the two curves coincide.
