Instant Maths Help:   07795 345383

Edexcel A Level

Locating Roots

Edexcel 9MA0/01 Jun 2024 A2 Exam Q. 3 a :   2 marks in 2:24 min.
Edexcel 9MA0/02 Jan 2023 A2 Mock Q. 2 b :   1 mark in 1:12 min.
Edexcel 9MA0/02 Jun 2019 A2 Shadow Exam Q. 11 b :   2 marks in 2:24 min.
Edexcel 9MA0/02 Jun 2019 A2 Exam Q. 11 b :   2 marks in 2:24 min.
Edexcel 9MA0/02 Jan 2019 A2 Mock Q. 7 a :   2 marks in 2:24 min.
Edexcel 9MA0/01 Jun 2018 A2 Exam Q. 4 a :   2 marks in 2:24 min.
Edexcel 9MA0/02 May 2018 A2 Mock Q. 6 c :   2 marks in 2:24 min.
Edexcel 9MA0/01 May 2017 A2 Sample Exam Q. 8 a :   2 marks in 2:24 min.
Zoom in on locating roots
Change of Sign - Zooming In to Find Roots
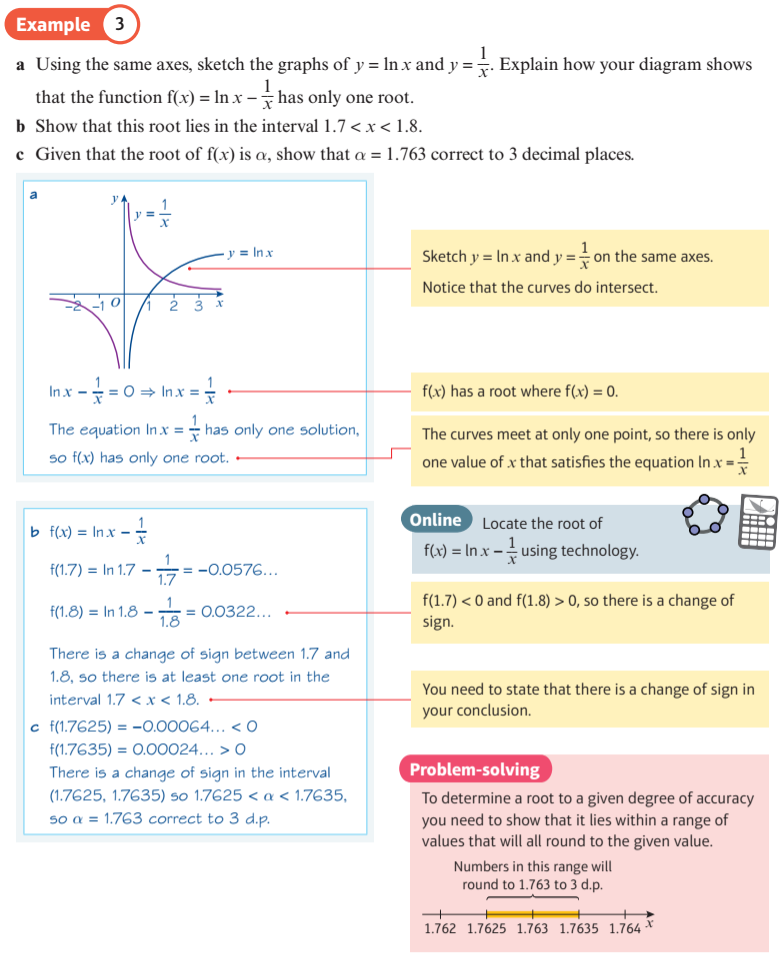
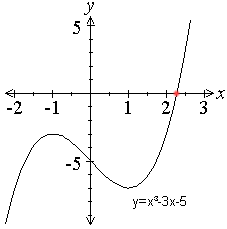change of sign Let \(f(x) = {x^3} - 3x - 5\).
To solve \({x^3} - 3x - 5 = 0\) , we need to find where the \(f(x)\) crosses the x-axis.

To do this we can display the graph \(f(x) = {x^3} - 3x - 5\) using computer software or a graphic calculator, and zoom in on the interval where the curve crosses the x-axis.

In the display below, you can use the navigation button to zoom in on the crossing point. A crossing point between x = 2.25 and x = 2.30 means the solution is 2.3 to 1 decimal place. As you zoom in further, you can narrow the limits of the solution further, and therefore give the solution with an increasing number of decimal places accuracy. Can you give the solution to 4 decimal places, or maybe 5?

After you have solved the initial problem, you can change coefficient b to 3 (for multiple solutions), and then change all the coefficients to investigate other cubic curves.
OPEN FULL-WINDOW
  Settings
Current A Level Syllabus
Current Stage
Current Calculator