Selected: Edexcel A Level Maths - Pure Maths
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
AQA 7357/3 Nov 2020 A2 Exam Q. 2 : 1 mark in 1:12 min.
AQA 7357/2 Jun 2019 A2 Exam Q. 6 : 6 marks in 7:12 min.
AQA 7357/1 Jun 2018 A2 Exam Q. 14 : 7 marks in 8:24 min.
OCR H240/02 Jun 2024 A2 Exam Q. 6 a : 5 marks in 6:00 min.
OCR H230/02 Jun 2024 AS Exam Q. 7 : 9 marks in 10:48 min.
OCR H240/01 Jun 2023 A2 Exam Q. 7 : 9 marks in 10:48 min.
OCR H240/02 Nov 2020 A2 Exam Q. 6 : 3 marks in 3:36 min.
OCR H240/03 Jun 2018 A2 Exam Q. 6 : 8 marks in 9:36 min.
OCR H240/03 Jun 2017 A2 Sample Exam Q. 3 : 4 marks in 4:48 min.
OCR H240/01 Jun 2017 A2 Sample Exam Q. 8 : 6 marks in 7:12 min.
Prove addition formulae using geometric construction
Visualise sin(2x) Double Angle Formulae
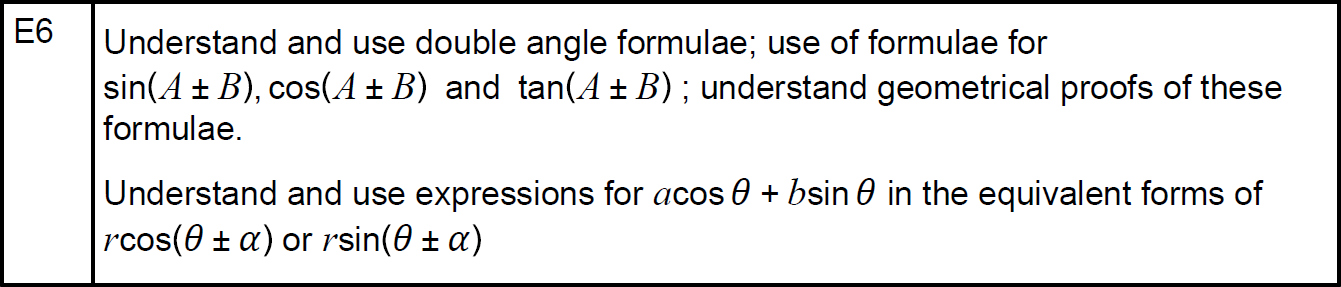
The double angle formulae are useful in solving trigonometric formulae.
This display allows you to view formulae relating to $\color{green}{\sin (a \, x)}$ with $\color{green}{a}$ an adjustable constant.
Initially, $\color{green}{a}$ is set to 0 so you can visualise how $\color{red}{\sin (c \, x) \cos (c \, x)}$ arises as the product of $\color{grey}{\sin (c \, x)}$ and $\color{grey}{\cos (c \, x)}$.
Then, by adjusting $\color{green}{a}$, $\color{red}{b}$ and $\color{red}{c}$ to make the green and red curves coincide, you can view the double angle formulae, not only for $\color{green}{\sin (2 \, x)}$, but for other values of $\color{green}{a}$ too.
Initially, $\color{green}{a}$ is set to 0 so you can visualise how $\color{red}{\sin (c \, x) \cos (c \, x)}$ arises as the product of $\color{grey}{\sin (c \, x)}$ and $\color{grey}{\cos (c \, x)}$.
Then, by adjusting $\color{green}{a}$, $\color{red}{b}$ and $\color{red}{c}$ to make the green and red curves coincide, you can view the double angle formulae, not only for $\color{green}{\sin (2 \, x)}$, but for other values of $\color{green}{a}$ too.
Visualise cos(2x) Double Angle Formulae
The double angle formulae are useful in solving trigonometric formulae.
This display allows you to view formulae relating to $\color{green}{\cos (a \, x)}$ with $\color{green}{a}$ an adjustable constant.
By adjusting $\color{green}{a}$, $\color{red}{b}$, $\color{red}{c}$ and $\color{red}{d}$ to make the green and red curves coincide, you can view the double angle formulae, for $\color{green}{\cos (2 \, x)}$ in its different forms.
By adjusting $\color{green}{a}$, $\color{red}{b}$, $\color{red}{c}$ and $\color{red}{d}$ to make the green and red curves coincide, you can view the double angle formulae, for $\color{green}{\cos (2 \, x)}$ in its different forms.
Visualise sin(x ${ \pm }$ a) Addition Formulae
This display allows you to visualise the addition formulae:
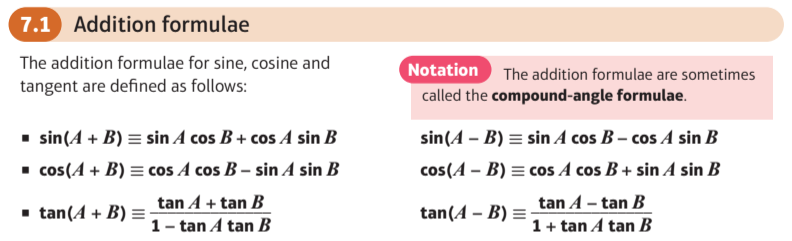
$\color{purple}{ \sin (x + a) = \sin(x) \cos(a) + \cos(x) \sin(a) }$
$\color{purple}{ \sin (x - a) = \sin(x) \cos(a) - \cos(x) \sin(a) }$
$\color{purple}{ \sin (x + a) = \sin(x) \cos(a) + \cos(x) \sin(a) }$
$\color{purple}{ \sin (x - a) = \sin(x) \cos(a) - \cos(x) \sin(a) }$
Visualise cos(x ${ \pm }$ a) Addition Formulae
This display allows you to visualise the addition formulae:
$\color{purple}{ \cos (x + a) = \cos(x) \cos(a) - \sin(x) \sin(a) }$
$\color{purple}{ \cos (x - a) = \cos(x) \cos(a) + \sin(x) \sin(a) }$
$\color{purple}{ \cos (x + a) = \cos(x) \cos(a) - \sin(x) \sin(a) }$
$\color{purple}{ \cos (x - a) = \cos(x) \cos(a) + \sin(x) \sin(a) }$
Visualise a sin x ${ \pm }$ b cos x to sin
This display allows you to visualise the transformations:
$\color{purple}{ a \, \sin (x) + b \, \cos (x) = R \, \sin (x + \alpha) }$
$\color{purple}{ a \, \sin (x) - b \, \cos (x) = R \, \sin (x - \alpha) }$
After adjusting $\color{red}{a}$ and $\color{blue}{b}$, you can adjust $\color{green}{r}$ and $\color{green}{\alpha}$ to their derived values to see the two curves coincide.
For a better view, you should use the navigation buttons in the lower right corner to zoom in and out.
$\color{purple}{ a \, \sin (x) + b \, \cos (x) = R \, \sin (x + \alpha) }$
$\color{purple}{ a \, \sin (x) - b \, \cos (x) = R \, \sin (x - \alpha) }$
After adjusting $\color{red}{a}$ and $\color{blue}{b}$, you can adjust $\color{green}{r}$ and $\color{green}{\alpha}$ to their derived values to see the two curves coincide.
For a better view, you should use the navigation buttons in the lower right corner to zoom in and out.
Visualise a cos x ${ \pm }$ b sin x to cos
This display allows you to visualise the transformations:
$\color{purple}{ a \, \cos (x) + b \, \sin (x) = R \, \cos (x - \alpha) }$
$\color{purple}{ a \, \cos (x) - b \, \sin (x) = R \, \cos (x + \alpha) }$
After adjusting $\color{red}{a}$ and $\color{blue}{b}$, you can adjust $\color{green}{r}$ and $\color{green}{\alpha}$ to their derived values to see the two curves coincide.
For a better view, you should use the navigation buttons in the lower right corner to zoom in and out.
$\color{purple}{ a \, \cos (x) + b \, \sin (x) = R \, \cos (x - \alpha) }$
$\color{purple}{ a \, \cos (x) - b \, \sin (x) = R \, \cos (x + \alpha) }$
After adjusting $\color{red}{a}$ and $\color{blue}{b}$, you can adjust $\color{green}{r}$ and $\color{green}{\alpha}$ to their derived values to see the two curves coincide.
For a better view, you should use the navigation buttons in the lower right corner to zoom in and out.
sin(x + a) + sin(x - a)
The display shows \(\color{blue}{y = \sin x}\) in blue and \(\color{red}{y = \sin \left( {x + a} \right) + \sin \left( {x - a} \right)}\)