Instant Maths Help:   07795 345383

OCR MEI A Level

Transformations

OCR MEI H640/02 Jun 2024 A2 Exam Q. 2 :   2 marks in 2:24 min.
OCR MEI H630/01 Jun 2023 AS Exam Q. 9 :   9 marks in 11:34 min.
OCR MEI H630/01 Jun 2022 AS Exam Q. 5 :   6 marks in 7:43 min.
OCR MEI H640/02 Nov 2021 A2 Exam Q. 1 :   2 marks in 2:24 min.
OCR MEI H640/01 Jun 2019 A2 Exam Q. 10 b :   3 marks in 3:36 min.
OCR MEI H640/03 Jun 2019 A2 Exam Q. 2 :   5 marks in 8:00 min.
OCR MEI H640/03 Jun 2018 A2 Exam Q. 2 :   2 marks in 3:12 min.
OCR MEI H630/01 Jun 2018 AS Exam Q. 9 :   9 marks in 11:34 min.
OCR MEI H640/02 Jun 2017 A2 Exam Q. 2 :   4 marks in 4:48 min.
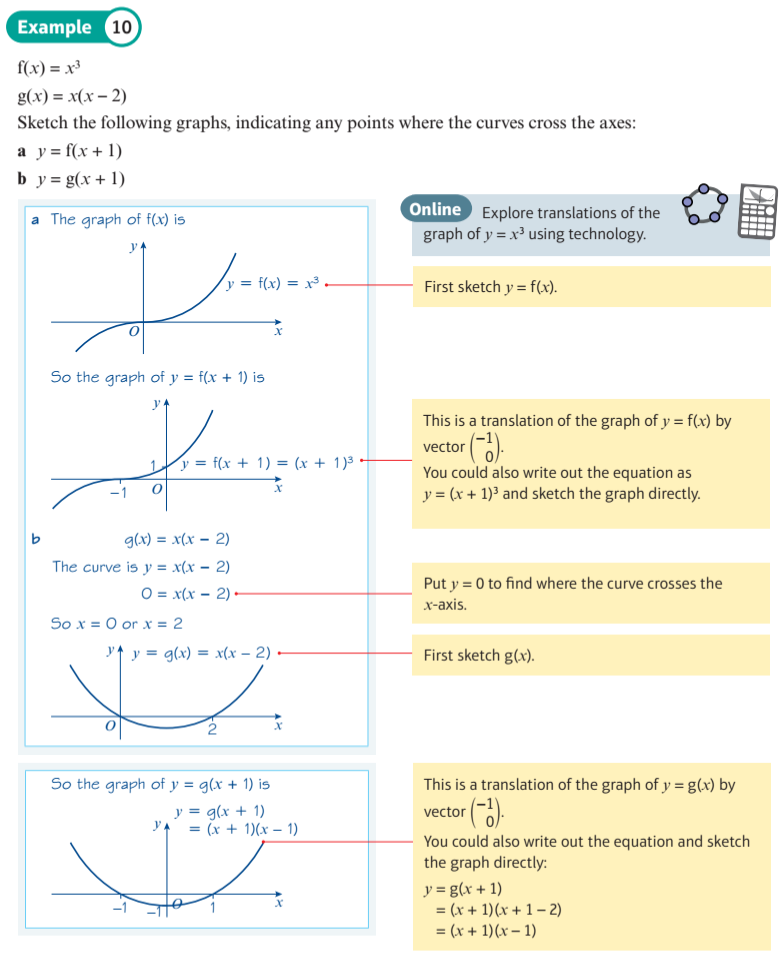
Explore translations of a graph
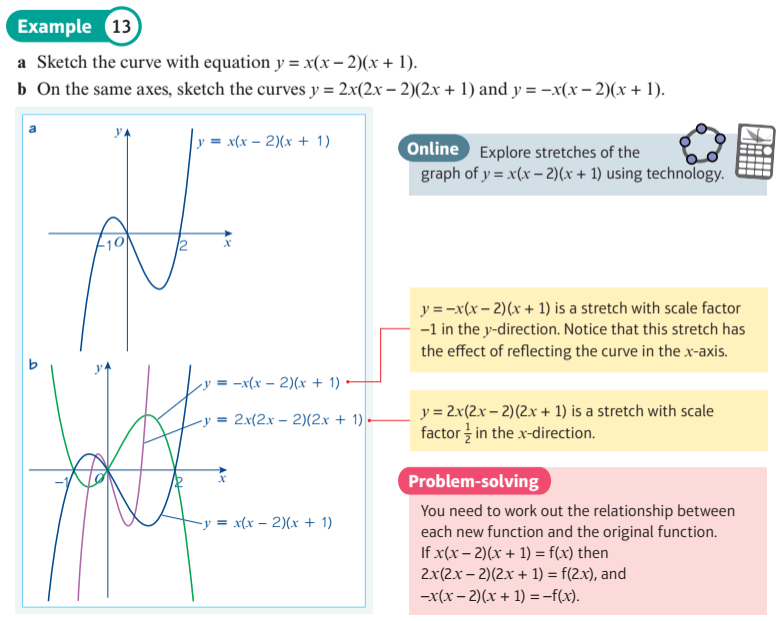
Explore stretches of a cubic graph
Explore transformations of exponential function
Explore combined tranformations of logarithms
  • a f(x) (AS) a f(x) (AS)
  • f(x + a) (AS) f(x + a) (AS)
  • f(x - a) (AS) f(x - a) (AS)
  • f(x) + a (AS) f(x) + a (AS)
  • f(a x) (AS) f(a x) (AS)
  • Combinations (A2) Combinations (A2)
  • sin Transf sin Transf
  • cos Transf cos Transf
  • tan Transf tan Transf
  • Order 1 (A2) Order 1 (A2)
  • Order 2 (A2) Order 2 (A2)
Transformation: y = a f(x)
For each example, investigate the effect on the graph of y = af(x) of changing 'a'.
A transformation may look like a rotation, but the draggable green point, shows they are all, in fact, stretches away from the x-axis by a factor of a.
What is the effect of making a = -1, so y = -f(x) ?
OPEN FULL-WINDOW
Transformation: y = f(x + a)
For each example, investigate the effect on the graph of y = f(x + a) of changing 'a'.
The green sample points show how graphs are translated horizontally in the opposite direction to what you might expect.
OPEN FULL-WINDOW
Transformation: y = f(x - a)
For each example, investigate the effect on the graph of y = f(x - a) of changing 'a'.
The green sample points show how graphs are translated horizontally.
OPEN FULL-WINDOW
Transformation: y = f(x) + a
For each example, investigate the effect on the graph of y = f(x) + a of changing 'a'.
You can drag the green point, and then use the slider to see the effect of the transformation on that particular point.
OPEN FULL-WINDOW
Transformation: y = f(a x)
For each example, investigate the effect on the graph of y = f(ax) of changing 'a'.
The green sample points show how graphs expand or contract horizontally in the opposite directions to what you might expect.
What is the effect of making a = -1, so y = f(-x) ?
OPEN FULL-WINDOW
Exponential and Log Combined Transformations
Two transformations of \(y = f\left( x \right)\) are considered: \(y = f\left( {ax} \right)\) and \(y = f\left( {x - b} \right)\) , together with their combination.

This display shows the transformation \(y = \ln \left( {ax} \right)\) What is the effect of increasing \(a\)? It should be a stretch factor \({1 \over a}\), but it looks like a translation. Noting that \(\ln \left( {ax} \right) = \ln \left( x \right) + \ln \left( a \right)\), you can see that the transformation is the same as a translation vertically by \(y = \ln \left( {a} \right)\). When \(a = - 1\), the transformation is a reflection in the \(y\) axis.

This display shows the transformation \(y = \ln \left( {x - b} \right)\) Increasing the value of \(a\) will move the curve to the right.

This display shows the transformations \(y = \ln \left( {{ax} - b} \right)\)
OPEN FULL-WINDOW
a sin (b x + c) + d Transformations
This display allows you to view transformations of the $\color{blue}{ \sin }$ function.

The original sin function is showed as a green dashed line, and the transformed sin function is shown as a solid blue line. Initially the lines coincide. You can drag green and blue gliders to read coordinates at corresponding points on the original and transformed curves.
OPEN FULL-WINDOW
a cos (b x + c) + d Transformations
This display allows you to view transformations of the $\color{blue}{ \cos }$ function.

The original cos function is showed as a green dashed line, and the transformed cos function is shown as a solid blue line. Initially the lines coincide. You can drag green and blue gliders to read coordinates at corresponding points on the original and transformed curves.
OPEN FULL-WINDOW
a tan (b x + c) + d Transformations
This display allows you to view transformations of the $\color{blue}{ \tan }$ function.

The original tan function is showed as a green dashed line, and the transformed tan function is shown as a solid blue line. Initially the lines coincide. You can drag green and blue gliders to read coordinates at corresponding points on the original and transformed curves.
OPEN FULL-WINDOW
Investigate Order of Transformations 1
This display shows the order in which transformations are applied generally makes a difference.
For $\color{blue}{f(x) = ( a \, \sin ( x ) ) + d }$ the vertical stretch (factor $\color{purple}{ a }$) is applied before the vertical translation (of $\color{purple}{ d }$).
For $\color{red}{g(x) = a \, ( \sin ( x ) + d ) }$ the vertical translation (of $\color{purple}{ d }$) is applied before the vertical stretch (factor $\color{purple}{ a }$).

You can drag a glider on the x-axis to help read cooordinates at corresponding sample points.
OPEN FULL-WINDOW
Investigate Order of Transformations 2
This display shows the order in which transformations are applied generally makes a difference.
For $\color{blue}{f(x) = \sin ( ( b \, x ) + c ) }$ the horizontal translation (of $\color{purple}{ - c }$) is applied before the horizontal stretch (factor $\color{purple}{ { 1 \over b } }$).
For $\color{red}{g(x) = \sin ( b ( x + c ) ) }$ the the horizontal stretch (factor $\color{purple}{ { 1 \over b } }$) is applied before the horizontal translation (of $\color{purple}{ - c }$).
These orders may seem counter-intuitive if considering 'BIDMAS' precedence.

You can drag a glider on the x-axis to help read cooordinates at corresponding sample points.
OPEN FULL-WINDOW
  Settings
Current A Level Syllabus
Current Stage
Current Calculator