Explore the general form of the equation of a circle
How many possible points of intersection between straight lines and circles ?
Tangent and chord properties
Explore the circumcircle of a triangle
Investigate Equation of Circle
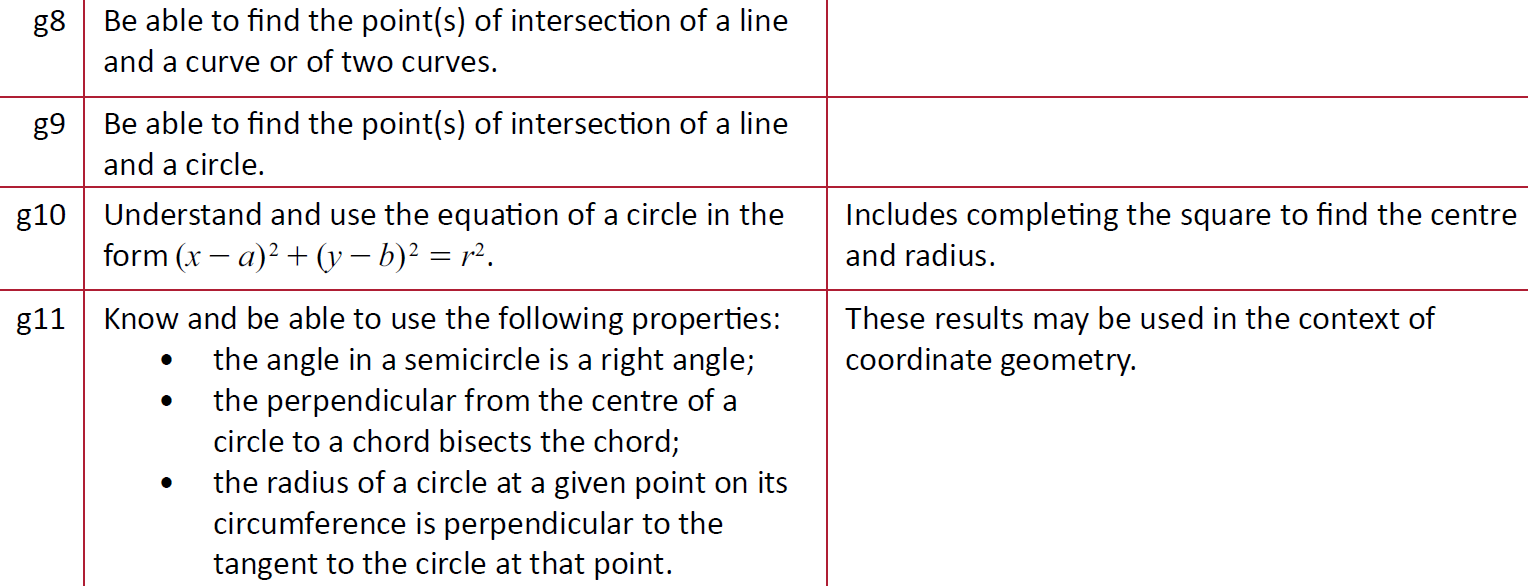
A circle can be expressed with the cartesian equation \(\color{blue}{{\left( {x - a} \right)^2} + {\left( {y - b} \right)^2} = {r^2}}\),
where a, b and r are constants.
The circle is centred at \(\color{blue}{(a, b)}\) and has radius \(\color{blue}{r}\).
where a, b and r are constants.
The circle is centred at \(\color{blue}{(a, b)}\) and has radius \(\color{blue}{r}\).
Matching Circles
You can select eight green circles on this page. Using the sliders to match the blue dashed circle to the green circle
will reveal the equation in each case, but can you say what the equation is before making the match?
