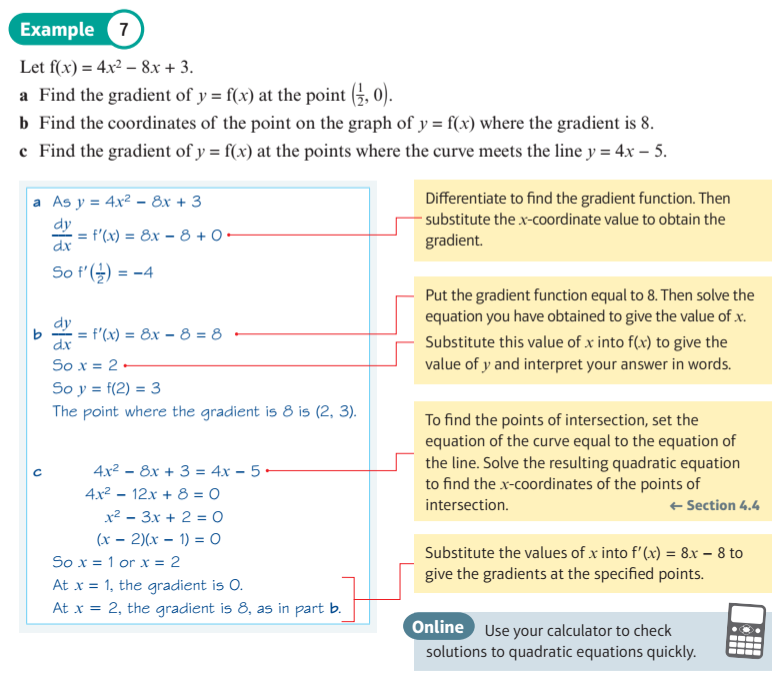
Checking solutions to quadratic equations in differentiation
1st and 2nd Derivatives of Composite Functions
This display allows you to investigate the first and second derivatives of some composite functions.
Visualise the Product Rule
The function $ {x ^ 2} \, (x−1) $ is a product of $ {x ^ 2} $ and $ (x−1) $.
The derivative of $ {x ^ 2} $ is $ 2x $ and the derivative of $ (x−1) $ is $ 1 $.
So, is the derivative of $ {x ^ 2} \, (x−1) $ equal to $ 2x(1) = 2x $, or $ 2x + 1 $, or something else?
This display allows you to visualise the curve $ {x ^ 2} \, (x − 1) $, as well as many other products of functions. You can use it to visualise the "Product Rule" which defines how gradient functions are derived for products of functions.
This display allows you to visualise the curve $ {x ^ 2} \, (x − 1) $, as well as many other products of functions. You can use it to visualise the "Product Rule" which defines how gradient functions are derived for products of functions.
Visualise the Quotient Rule
The connection between a function $ h(x) $ and it derivative $ h'(x) $ is much harder to see when the function
$ h(x) $ is a quotient of two other functions.
This display allows you to visualise the curve $ { x \over x + 1 } $, as well as many other quotients of functions. You can use it to visualise the "Quotient Rule" which defines how gradient functions are derived for quotients of functions.
This display allows you to visualise the curve $ { x \over x + 1 } $, as well as many other quotients of functions. You can use it to visualise the "Quotient Rule" which defines how gradient functions are derived for quotients of functions.
