Selected: Edexcel A Level Maths - Pure Maths
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
AS & A2 (Whole Course) - Casio fx-991EX
Register / Login for More / Subscribe for All Without Ads
OCR (A) H240/01 Jun 2023 A2 Exam Q. 3 : 7 marks in 8:24 min.
OCR (A) H230/02 Jun 2022 AS Exam Q. 8 a : 4 marks in 4:48 min.
OCR (A) H230/02 Nov 2021 AS Exam Q. 4 : 5 marks in 6:00 min.
OCR (A) H240/02 Nov 2021 A2 Exam Q. 7 : 4 marks in 4:48 min.
OCR (A) H240/01 Jun 2019 A2 Exam Q. 6 : 6 marks in 7:12 min.
OCR (A) H230/02 Jun 2017 AS Sample Exam Q. 7 : 5 marks in 6:00 min.
Edexcel 9MA0/01 Jun 2023 A2 Exam Q. 12 : 5 marks in 6:00 min.
Edexcel 9MA0/02 Jun 2022 A2 Exam Q. 4 : 3 marks in 3:36 min.
Edexcel 8MA0/01 Nov 2021 AS Exam Q. 5 : 6 marks in 7:12 min.
Edexcel 9MA0/01 Jan 2019 A2 Mock Q. 5 : 5 marks in 6:00 min.
Edexcel 8MA0/01 Jun 2018 AS Exam Q. 10 : 4 marks in 4:48 min.
Edexcel 9MA0/02 Jun 2018 A2 Exam Q. 9 : 5 marks in 6:00 min.
Edexcel 9MA0/01 May 2018 A2 Mock Q. 9 : 5 marks in 6:00 min.
Edexcel 8MA0/01 Jun 2017 AS Sample Exam Q. 6 : 4 marks in 4:48 min.
Edexcel 9MA0/01 May 2017 A2 Sample Exam Q. 10 : 5 marks in 6:00 min.
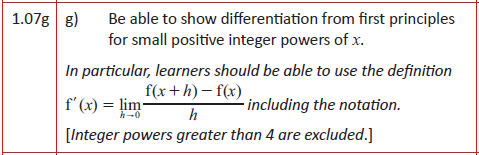
Derivative as limit of chord gradient
Differentiating Quadratics
At any point on a curve, a line can be drawn that just touches the curve at that point.
Such a line is called a tangent. This tangent line will have a gradient.
Drag the red point in the display below to move the tangent line along the quadratic graph, and see how the gradient changes.
You can see that the gradient function f'(x) of a quadratic curve is a straight line.
Drag the red point in the display below to move the tangent line along the quadratic graph, and see how the gradient changes.
You can see that the gradient function f'(x) of a quadratic curve is a straight line.
Differentiating Cubics
At any point on a curve, a line can be drawn that just touches the curve at that point.
Such a line is called a tangent. This tangent line will have a gradient.
Drag the red point in the display below to move the tangent line along the cubic graph, and see how the gradient changes.
You can see that the gradient function f'(x) of a cubic curve is a quadratic curve.
Drag the red point in the display below to move the tangent line along the cubic graph, and see how the gradient changes.
You can see that the gradient function f'(x) of a cubic curve is a quadratic curve.
Differentiating Quartics and Higher Order
Drag the red point in the display below to move the tangent line along the quartic (fourth order) curve.
You can see that the gradient function f'(x) of a quartic curve is a cubic curve.
Finally you can investigate higher order curves. In each case, the gradient function f'(x) will be a curve of one order lower.
You can see that the gradient function f'(x) of a quartic curve is a cubic curve.
Finally you can investigate higher order curves. In each case, the gradient function f'(x) will be a curve of one order lower.
Gradients of Polynomials Including Negative Indices
Use this display to investigate the gradient functions of your own polynomials.
You can also set coefficients of negative indices \(x^{-1}\), \(x^{-2}\) and \(x^{-3}\).
Drag the red sample point to help you investigate.
You can also set coefficients of negative indices \(x^{-1}\), \(x^{-2}\) and \(x^{-3}\).
Drag the red sample point to help you investigate.
Differentiating ${e^x}$ and ${ln (x)}$
Use this display to investigate f(x) and f'(x) for ${e^x}$ and ${ln (x)}$.
You can change f(x) with the sliders and drag the red sample point.
You can change f(x) with the sliders and drag the red sample point.
Chords and Tangents of Polynomials
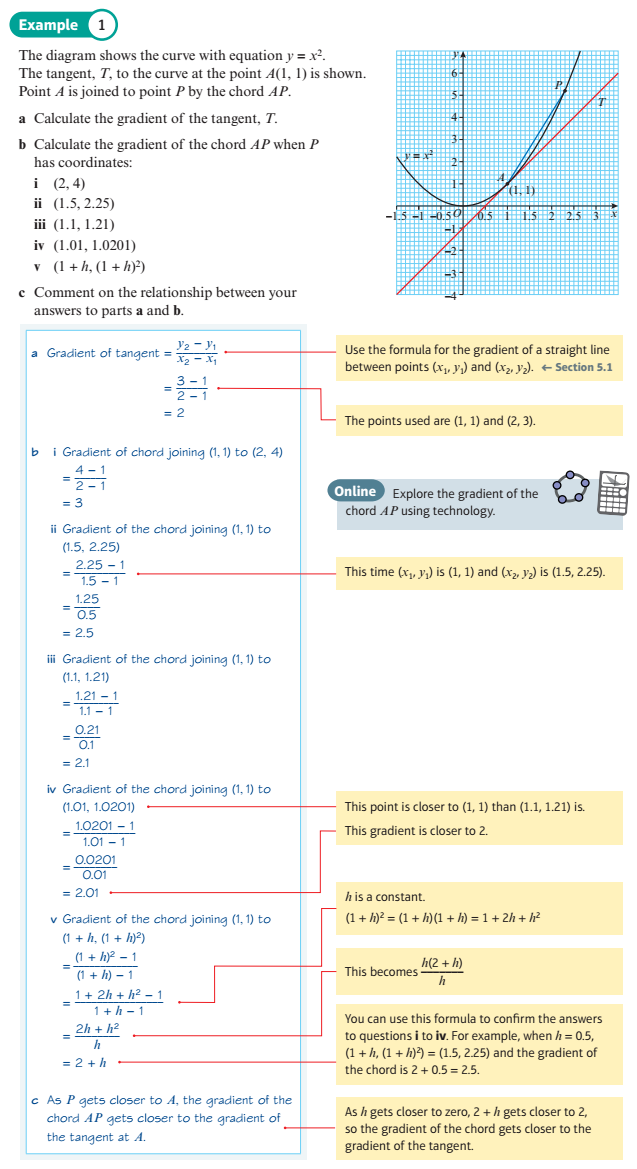
You can use this display to see how the gradient of a chord (or secant) approaches
the gradient of a tangent as the length of the chord approaches zero.
Having defined your polynomial, with the blue sliders, you can set the tangent point with the "tangent x" slider, and then drag an approaching point towards it with the "approach x" slider.
Having defined your polynomial, with the blue sliders, you can set the tangent point with the "tangent x" slider, and then drag an approaching point towards it with the "approach x" slider.
Chords and Tangents of Trig Functions
You can use this display to see how the gradient of a chord (or secant) approaches
the gradient of a tangent as the length of the chord approaches zero.
Having selected your trigonometric function, you can set the tangent point with the "tangent x" slider,
Having selected your trigonometric function, you can set the tangent point with the "tangent x" slider,
Investigate Differentiation of ${ln (ax)}$
Use this display to investigate the gradient, ie derivative, of $\color{blue}{ f(x) = \ln \left( a \, x \right) }$,
as a changes.
as a changes.
Investigate Trig Differentiation
This display allows you to investigate the first and second derivatives of standard trigonometric functions.
The graph of the first derivative is shown in red and the graph of the second derivative is in purple.
The x-slider allows you to run along the curves to see correpondence at a single point.
Can you see how the derivative of sin is cos?
Can you see how the derivative of cos is -sin?
Can you see how the derivative of tan is sec2?
The graph of the first derivative is shown in red and the graph of the second derivative is in purple.
The x-slider allows you to run along the curves to see correpondence at a single point.
Can you see how the derivative of sin is cos?
Can you see how the derivative of cos is -sin?
Can you see how the derivative of tan is sec2?
Derivatives of Inverses arcsin, arccos, arctan
This display allows you to investigate the derivatives of the inverse trigonometric functions $\color{blue}{\arcsin (x)}$, $\color{blue}{\arccos (x)}$ and $\color{blue}{\arctan (x)}$.