Instant Maths Help:   07795 345383

OCR A Level Maths - Gradient Problems

Differentiation Maxima, Minima & Inflection

OCR (A) H240/01 Jun 2024 A2 Exam Q. 11 :   12 marks in 14:24 min.
OCR (A) H230/01 Jun 2024 AS Exam Q. 5 :   9 marks in 10:48 min.
OCR (A) H240/02 Jun 2023 A2 Exam Q. 4 :   9 marks in 10:48 min.
OCR (A) H240/02 Jun 2023 A2 Exam Q. 5 :   12 marks in 14:24 min.
OCR (A) H230/01 Jun 2023 AS Exam Q. 6 bc :   8 marks in 9:36 min.
OCR (A) H240/02 Jun 2022 A2 Exam Q. 5 :   4 marks in 4:48 min.
OCR (A) H230/01 Nov 2021 AS Exam Q. 2 :   4 marks in 4:48 min.
OCR (A) H240/02 Nov 2021 A2 Exam Q. 4 :   10 marks in 12:00 min.
OCR (A) H230/01 Nov 2021 AS Exam Q. 5 :   8 marks in 9:36 min.
OCR (A) H240/01 Nov 2021 A2 Exam Q. 5 b :   3 marks in 3:36 min.
OCR (A) H240/01 Nov 2020 A2 Exam Q. 3 :   10 marks in 12:00 min.
OCR (A) H240/03 Nov 2020 A2 Exam Q. 4 a :   5 marks in 6:00 min.
OCR (A) H230/02 Nov 2020 AS Exam Q. 7 :   13 marks in 15:36 min.
OCR (A) H230/02 Jun 2019 AS Exam Q. 2 :   6 marks in 7:12 min.
OCR (A) H240/02 Jun 2019 A2 Exam Q. 5 :   9 marks in 10:48 min.
OCR (A) H240/02 Jun 2018 A2 Exam Q. 6 :   13 marks in 15:36 min.
OCR (A) H240/02 Jun 2017 A2 Sample Exam Q. 2 :   7 marks in 8:24 min.
OCR (A) H230/01 Jun 2017 AS Sample Exam Q. 4 :   7 marks in 8:24 min.
OCR (A) H240/03 Jun 2017 A2 Sample Exam Q. 6 :   7 marks in 8:24 min.
Finding value of 1st derivative at given point
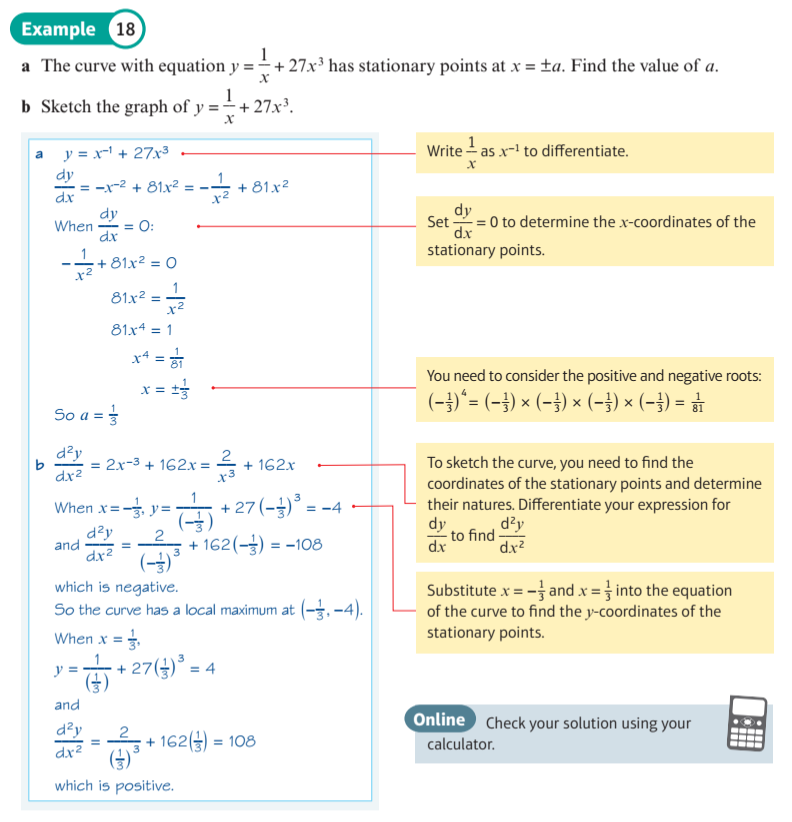
Explore tangent and normal to curve
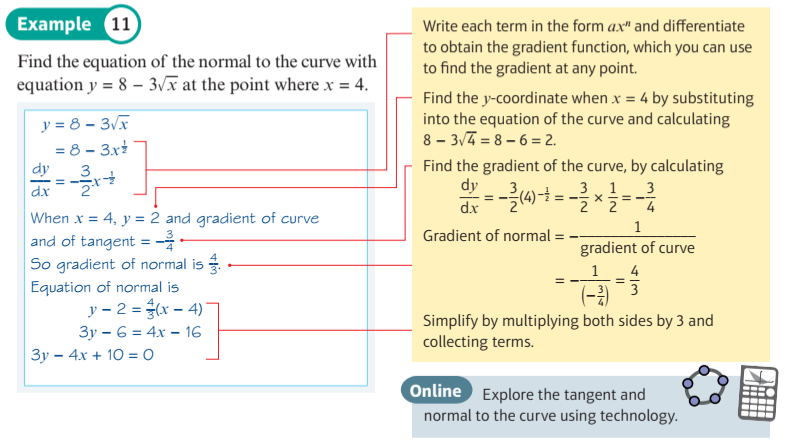
Explore where function is increasing and decreasing
Explore a stationary point
Explore key features linking function and derivative
Explore the derivatives of sin and cos
Explore derivative of a to power x
Exploring second derivatives
1st and 2nd Derivatives
This display allows you to investigate first and second derivatives using polynomial functions. When you drag the blue point on the polynomial curve at the top you should see the corresponding first and second derivative curves lining up below it. The first derivative is the gradient function, and the second derivative is the gradient of the gradient.

Can you see how the second derivative of a maximum point is negative, and that of a minimum point is positive?

Can you see how the second derivative of a point of inflection is zero?
OPEN FULL-WINDOW
Stationary Points - Maxima and Minima
Look carefully at the points on the graph of y = f(x) where the curve becomes horizontal. What is the value of f'(x) at these points?

These points are called stationary points. The "top of the hill" of the curve is called a maximum point. The "bottom of the valley" of the curve is called a minimum point.

You can drag the blue sample point to help you investigate.
OPEN FULL-WINDOW
Stationary Points - Maxima and Minima
Tangent and normal lines to the graph of $y = f(x)$ are displayed in green and red respectively.
A sample point, which you can drag to help investigate, is displayed in blue.

Look carefully at the points where the curve becomes horizontal. What is the value of x at these points? These points are called stationary points. The "top of the hill" of the curve is called a maximum point. The "bottom of the valley" of the curve is called a minimum point.

Can you find those points where the gradient is at its greatest? And where the gradient is at its smallest?
OPEN FULL-WINDOW
Points of Inflection
Look carefully at the points on the graph of y = f(x) where the curve becomes horizontal. f'(x) = 0 at these points. Many of these stationary points are either maximum or minimum points, but there are a few that are neither, for example at x = -1.57 and 4.71. These are called points of inflexion.

You can drag the blue sample point to help you investigate.
In the display below the graph of y = f(x) is shown for polynomial functions, together with the graphs of y = f'(x) and y = f''(x).

f(x) is initialised to $y = {\left( {x + 1} \right)^3} + 3$ $(= {x^3} - 3{x^2} + 3x + 2)$
This curve has a point of inflexion at x = 1. What are the values of f'(x) and f''(x) at this point?

Once again, you can drag the blue sample point to help you investigate. You can then check your findings with curves of your own.
OPEN FULL-WINDOW
An Increasing Function
The function $f\left( x \right) = {x^3} + x$ is always increasing. You can see that its gradient is never negative.

The function $f\left( x \right) = {x^3}$ has a gradient of zero at $x = 0$
OPEN FULL-WINDOW
A Decreasing Function
The function \(f\left( x \right) = 1 - x - {x^3}\) is always decreasing; its gradient is always negative.
OPEN FULL-WINDOW
Increasing or Decreasing?
Identify which of the following 10 curves show increasing or decreasing functions, or neither.
OPEN FULL-WINDOW
Strictly Increasing/Decreasing Functions
This display shows the difference between increasing/decreasing and strictly increasing/decreasing functions.

The gradient of $f(x) = {x^3} + 1$ is 0 at $x = 0$, but it is still strictly increasing at this point, because to the left $f(x)$ is less, and to the right $f(x)$ is more ($\forall a,b:a < b \Rightarrow f(a) < f(b)$).

The gradient of $f(x) = - {x^3} + 1$ is 0 at $x = 0$, but it is still strictly decreasing at this point, because to the left $f(x)$ is more, and to the right $f(x)$ is less ($\forall a,b:a < b \Rightarrow f(a) > f(b)$).
OPEN FULL-WINDOW
  Settings
Current A Level Syllabus
Current Stage
Current Calculator